Pulleys (Solutions)
The following solutions are for the problems on pulleys in the context of mechanical equilibrium linked here. I encourage you to attempt them by yourself first before looking through the solutions.
Problem 1
Consider the following system with negligible friction and a massless rope.

Find the acceleration and force acting on both of these objects. Be sure to include the directions of the vectors as well.
These two blocks are connected by a single rope, so we know that the magnitude of their accelerations $a$ is the same. Because there is no friction and no external forces other than gravity, we know that $m$ will fall downwards and $M$ will accelerate to the right in the $+\hat{x}$ direction. Mass $m$ has two forces acting on it: tension $T\hat{y}$ and gravity $-mg\hat{y}$, so using Newton’s second law on mass $m$, we have
\[ma=mg-T\longrightarrow a=g-\frac{T}{m}\]Notice that the right hand side is $mg-T$ instead of $T-mg$ because we are assuming that the mass $m$ accelerates in the $-\hat{y}$ direction instead of the $+\hat{y}$ direction, so quantities in the $-\hat{y}$ direction should be treated as “positive.” Meanwhile, mass $M$ only has one force acting on it: tension $T\hat{x}$, and so it’s second-law equation is
\[Ma=T\longrightarrow a=\frac{T}{M}\]Using the above two equations, we know have two equations for $a$, so equating them gives
\[g-\frac{T}{m}=\frac{T}{M}\longrightarrow g=T\frac{M+m}{Mm}\longrightarrow T=\frac{gMm}{M+m}\]Plugging this expression for the magnitude of the tension force $T$ into the second equation for $a$ above, we have
\[a=\frac{T}{M}=\frac{gm}{M+m}\]Therefore, we can conclude that the magnitude of the two relevant quantities in this problem $a$ and $T$ is
$$a=\frac{gm}{M+m}, \quad T=\frac{gMm}{M+m}$$
Problem 2
Consider the following system with negligible friction and a massless rope.
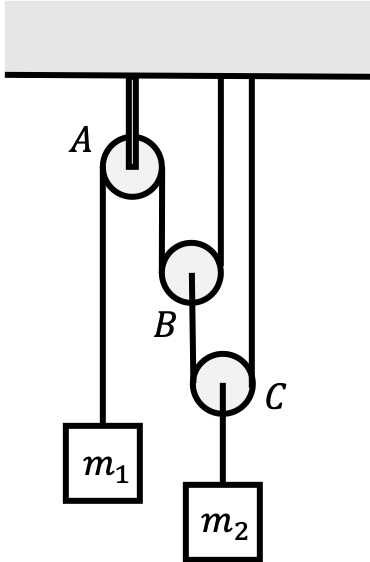
The mass of Pulley $A$ is $m_A$, the mass of Pulley $B$ is $m_B$, and the mass of Pulley $C$ is $m_C$. Find the condition on $m_2$ in terms of $m_1$ in order for the system to be in mechanical equilibrium.
As we will see, what appears to be a complicated setup of multiple pulleys is actually not too difficult to consider under the assumption that we want the system to be in mechanical equilibrium.
We’ll start with considering the force of tension $T$ in the $+\hat{y}$ direction acting on $m_1$. Because of the nature of pulleys, the segments of rope on both “sides” of Pulley $A$ also have tension with magnitude $T$, and so the rope connecting Pulleys $A$ and $B$ experiences a tension with magnitude $T$. By the nature of pulleys, this means that the segments of rope on both “sides” of Pulley $B$ also have a tension with magnitude $T$.
The tension $T_C$ acting on the two sides of the separate rope connected to Pulley $C$ is a bit more difficult to consider. To solve for $T_C$ as a function of $T$, we can use the equilibrium force balance condition on Pulley $B$. Accounting for all appropriate forces (two upwards tensions $T$, one downward tension $T_C$, and the force of gravity acting on $m_B$),
\[T+T-m_Bg-T_C=0\longrightarrow T_C=2T-m_Bg\]Of course, this is assuming that $m_Bg\leq 2T$. That is, we’ll assume that the mass $m_B$ of Pulley $B$ isn’t too heavy such that there’s no way it can be in mechanical equilibrium. Now, for the equilibrium condition on Pulley $C$, accounting for two upwards tensions $T_C$ and the forces of gravity acting on $m_2$ and $m_C$,
\[T_C+T_C-m_2g-m_Cg=2T_C-(m_C+m_2)g=0\]Plugging in our expression for $T_C$ in terms of $T$ from above,
\[4T-2m_Bg-m_2-m_Cg=0\]In order for mass $m_1$ to be in equilibrium, $T=m_1g$ necessarily, and so
\[4m_1g-2m_Bg-m_2-m_Cg=0\longrightarrow m_2g=4m_1g-2m_Bg-m_Cg\]Therefore, we can conclude that
$$m_2=4m_1-2m_B-m_C$$
Of course, this is assuming the appropriate relationship between $m_1, m_B, m_C$ such that $m_2>0$ (otherwise, our solution is not physically relevant).